documentation
PRODUCT SET UP
BLACK FOX PRO SET UP
BLACK FOX ONE SETUP
WHITE PAPERS
DESIGN FOR VIBRATION
A question I am often asked is how smooth is your grinder. The simple answer is that on its own it is very smooth. But the overall answer is a bit more complex. To understand the problem fully we need to take a look at the basics of design for vibrations.
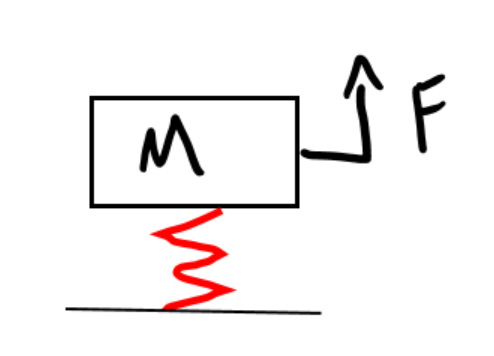
A basic vibratory system can be represented as:
Where we have a mass (M – this is the grinder), a forcing function (F – this is the motor and drive wheel), and a spring attached to the ground (this is your support structure). One practical example of this is a flagpole. You have some mass (M) on a structure with some spring constant value (k) and a force function (F) applied by wind. If you increase the mass at the top of the pole, the natural frequency of the pole will decrease making it more susceptible to vibrations by the forcing function (wind). The natural frequency (w) of any system can be found by w=sqrt(k/m).
In the case of a grinder or other piece of equipment, the system is essentially the same just a few more variables we have to consider. First is the center of gravity of the system. Second is the location of where the force is applied.
Let us look at a simplified grinder system of the grinder without any support structure. The motor and drive wheel are spinning at some RPM. The slight variations, due to manufacturing, will cause a force to act on the grinder about the center of gravity. This forcing function will grow based on how well the motor and the drive wheel are balanced. If you have a good motor and drive wheel the force should be very small. As the motor spins this small imbalance will impart a sinusoidal (alternating) force about the center of gravity of the grinder. The distance between the force and center of gravity will determine the amplitude of vibration. There are some small things you can do in your design to help dampen the perceived vibrations. One is to use a stiff lightweight material to dampen the vibrations thru it. Think of this material as a mini natural frequency calculation within the machine. If the natural frequency is high thru the material then it will be harder to transmit vibrations.
Now let us consider the grinder and its support structure. This example the same as the flagpole example earlier just slightly different. The grinder becomes the mass at the top of the flagpole. The support structure is your spring. The forcing function is the same as the simplified grinder system. As the k value (stiffness) of the support structure decreases so will the natural frequency. As the natural frequency of the support structure gets closer to the running frequency of the motor the more the system will vibrate with the amplitude applied by the forcing function. With a VFD the frequency of the motor can be varied from 0-58 Hz.
This is where design for vibrations can get interesting. Let say your structure had a natural frequency of 25 Hz. The system would vibrate the worst once the motor reaches 25 Hz but might be smooth at 0 Hz and 50 Hz. You can also get into more complex modes of natural frequency like; modes for bending in the x direction, bending in the y direction, and twisting. Those modes are usually out of the range of the system design unless the machine was designed poorly with a weak structure.
In conclusion, the Black Fox PRO and the Black Fox ONE uses high quality motors and drive wheels to mitigate the vibration amplitude, a stiff ½” drive plate, spacers that are plastic infused with carbon fiber to dampen vibrations, and good design practices for vibration mitigation. If the support structure is not built well then vibrations thru the complete system are still possible. If you are experiencing vibrations, I recommend increasing the stiffness of your support structure to eliminate unwanted vibrations.
PLATEN WATER-COOLING SYSTEM
DOWNLOAD THE WHITEPAPER
The water-cooling system for a platen pumps water into the top of the platen, where it then winds through to the bottom end. The water is then brought through the bottom wheel of the platen assembly and then through the top wheel. This helps to cool the parts with the highest dependence on temperature. Under high temperatures, the platen can cause contact burns if accidentally touched. Additionally, bearings can fail due to high temperatures. The water-cooling system will transfer heat from the platen and bearings so they remain at a safe temperature.
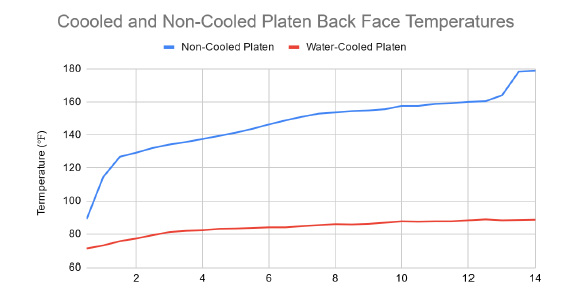
After 15 minutes with constant applied pressure to a 36 grit belt, a platen can can reach 180℉. OSHA states that metal surfaces subject to contact and user maintenance should have their temperature limited to 140℉ to protect workers from accidental contact burns. While using the water-cooling system, the platen temperature did not reach 90℉ after 15 minutes of use with a 36 grit belt.
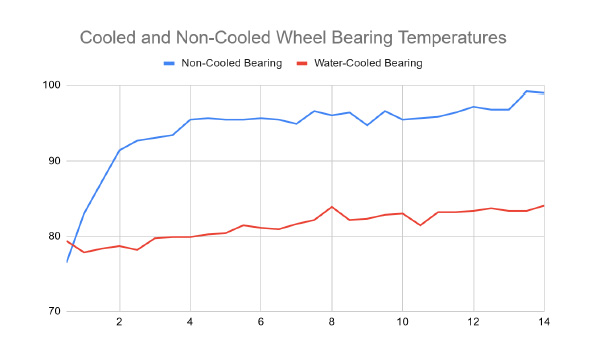
During the same test, the wheel bearings that were not water-cooled reached a temperature of 100℉. The failure rating of the bearing housing is 180℉, which was the maximum temperature of the nearby platen. When the water-cooling system was used, the bearing remained below 85℉.
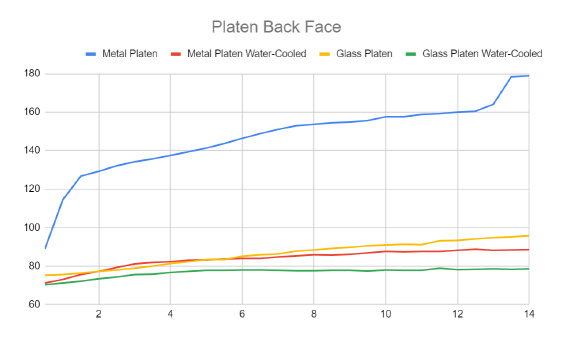
The water-cooling system can also be used with the glass platen attachment. The glass platen alone stays significantly more cool than the metal platen. After 15 minutes of constant applied pressure against a 36 grit belt, the glass platen’s max temperature was more than 80℉ less than the metal platen. This temperature difference can be again increased to 100℉ less than the metal platen by using the glass platen with the water-cooling system. During this experiment, the back face of the water-cooled glass platen remained consistent with the surrounding room temperature.